Lesson 2B: What are Limits?

What is a limit?

In math (and in life,) we sometimes want to describe what we are APPROACHING as time ticks along.   Who knows if we’ll ever get there, but it would be great to have a way to say, “Yup — we’re heading toward an output ( y ) value of two,” for example.

Maybe there’s a horizontal asymptote at y=2 (see below.)  Does the function ever actually get to touch y=2?   It does NOT, but it sure gets close!   In other words, the function is approaching y=2 as x values get really big (maybe even infinity?)

Check it out:
as x gets bigger and bigger, the y-values are approaching y=2.  (But they never actually get there!)

Here’s another example.

This function below has a hole in it (it is not continuous at x=2.).  Maybe the function has a divide by zero issue, causing a momentary hole in the graph.

As the graph APPROACHES x=2 from the right OR from the left, it sure looks like we’re heading for y=4, doesn’t it?
But does the “roller coaster” ever actually arrive at the point (2,4)?  NO!  The function doesn’t exist there.

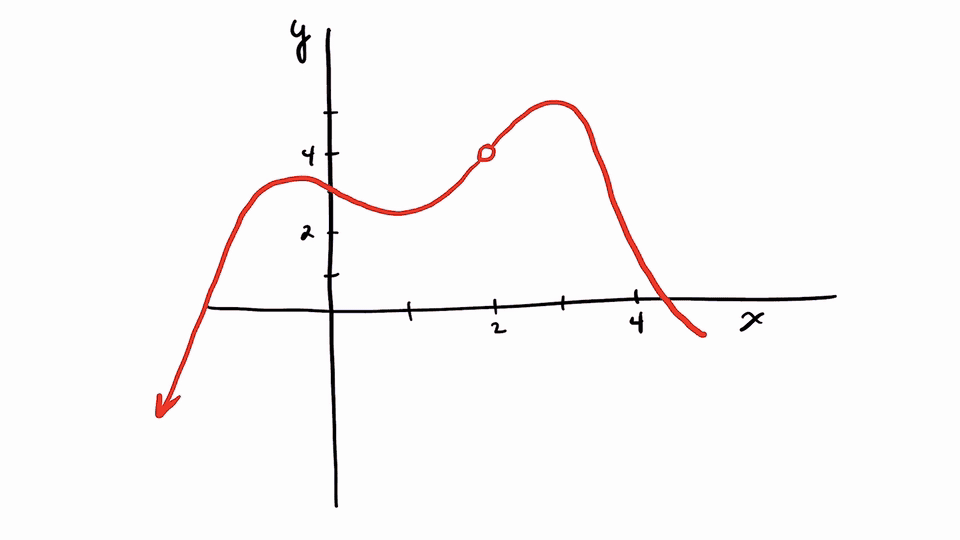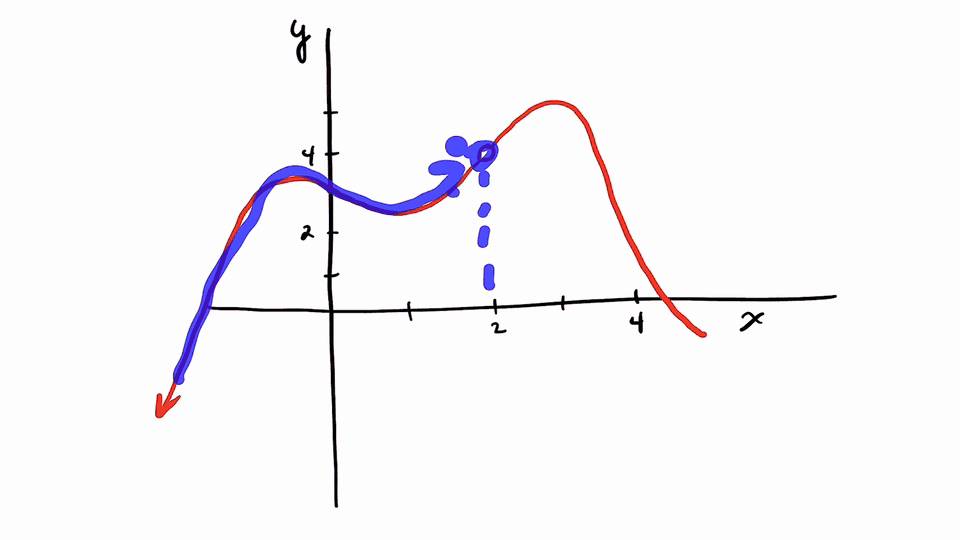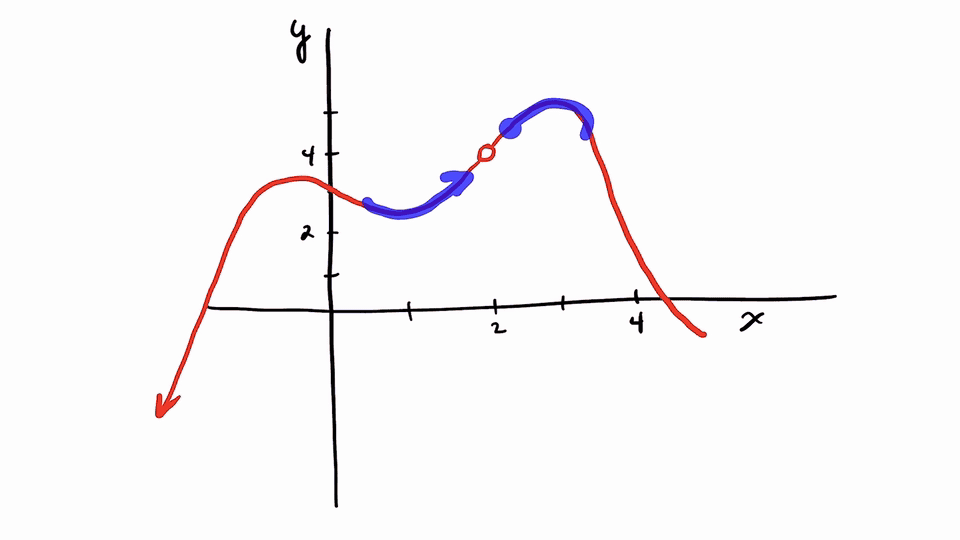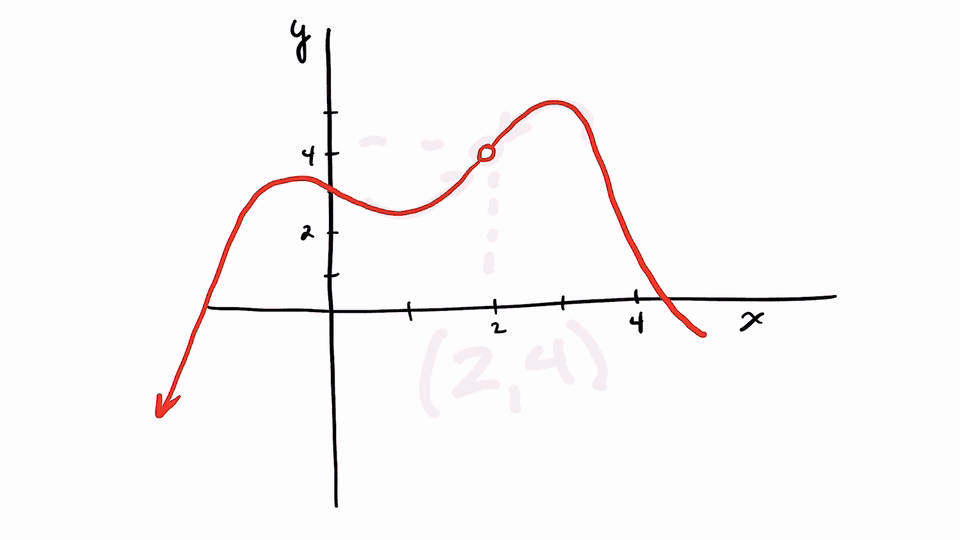
We would say that the limit as x approaches 2 is ….. FOUR!

If you think of x as being “time”, then you could imagine as we approach t=2, or the second minute of our experiment, the outputs are starting to get really close to 4.

The Notation of Limits: How do you write them?

I’ve added a bit to the graph now, so you can practice spotting values of limits.  We already said,

“As x approaches 2,  f (x) approaches 4″.

We can write that statement as a mathematical limit like this:

Read outloud:  “The limit as x approaches 2 on f (x) is 4″

Did you notice there is now an EQUAL sign?   You said, “IS FOUR” — not approaching four.  You see, a limit allows you to express a definitive value that the function IS approaching.   From the graph above, what are the values of these three limits?

Remember, the function doesn’t have to exist at the limit.

Why?  Because limits are all about what the output values are getting close to as you APPROACH an x value.   It’s NOT about whether the point exists or not.   Remember that open circle above at (4,2)?    The limit at x=4 was 2 even though the point (4,2) didn’t exist.

Here is an inline randomized question.  Some will have an open circle and some will be a closed circle.   Be sure to try enough to get a feel for the difference.  Keep trying until you’re confident.